How a mathematician dances
How Dance and Math are intertwined
Below is a video of a TED talk done by Dr. Karl Shaffer and Erik Stern. They teach math through dance movements at the Dr. Shaffer and Mr. Stern Dance Ensemble in Santa Cruz, California. They founded their Dance Ensemble in 1987. This was uploaded to the official TED talks youtube page on November 18, 2012. They gave the TED talk to expose people to how they teach math using movements to help people understand mathematical concepts such as symmetry through dance. Symmetry can be seen between two dancers as they dance next to each other on stage (Schaffer and Stern, n.d.).
Schaffer, K., & Stern, E. (n.d.). TEDxManhattanBeach. TEDxManhattanBeach. Retrieved from https://www.youtube.com/watch?v=Ws2y-cGoWqQ&feature=emb_logo
Below is an article called Mathematics in the World of Dance written by Katarzyna Wasilewska from the mathematics Department at the University of Southern California. In this academic resource, Wasilewska draws attention to the geometry that can be found in dance movements as well as how patterns can be seen in dance. Geometry can be found in the movements of individual dancers and in the formations made by groups of dancers. Patterns are used both in the choreography of pieces and in formations throughout a piece. Wasilewska presents factual information in an unbiased way throughout the journal.
Wasilewska, K. (n.d.). PDF. Los Angeles. Retrieved from https://hsbc.burlington-nj.net/ourpages/auto/2014/1/15/31986619/Mathematics%20in%20the%20World%20of%20Dance.pdf
Below is the link to an article written by Megan Scudellari called “Science and Culture: Dancing with Pythagoras”. This article is on the professional webpage, National Academy of Sciences of the United States of America. The article was published on March 22, 2016. The article does not contain bias. It explains the process for choreographing a routine called “Interpretations of the Pythagorean Problem” which used dancers to visually represent the pythagorean triangle and move in ways that come from equations mathematically derived from the triangle.
Here is a link to the performance https://vimeo.com/75623011. Here is an image depicting the paths the dancers travel throughout the piece.
Scudellari, M. (2016, March 22). Science and Culture: Dancing with Pythagoras. Retrieved November 16, 2019, from https://www.pnas.org/content/113/12/3123.
Sarah Goodman from MIT Student Life published a news article in the MIT news on February 24, 2016 about how dance is used to teach young girls math to help them gain confidence in their abilities. The program is called SHINE for girls and they explain equations such “4x+y” (Goodman, 2016) with the dance combination ball change, ball change, turn, ball change, ball change (Goodman, 2016). In the combination a ball change represents the variable x and the turn represents the variable y: this helps girls understand that x and y are different variables and must be thought of as different (Goodman, 2016). Goodman explains the program’s goal, how they got started, and techniques used to teach math to the girls.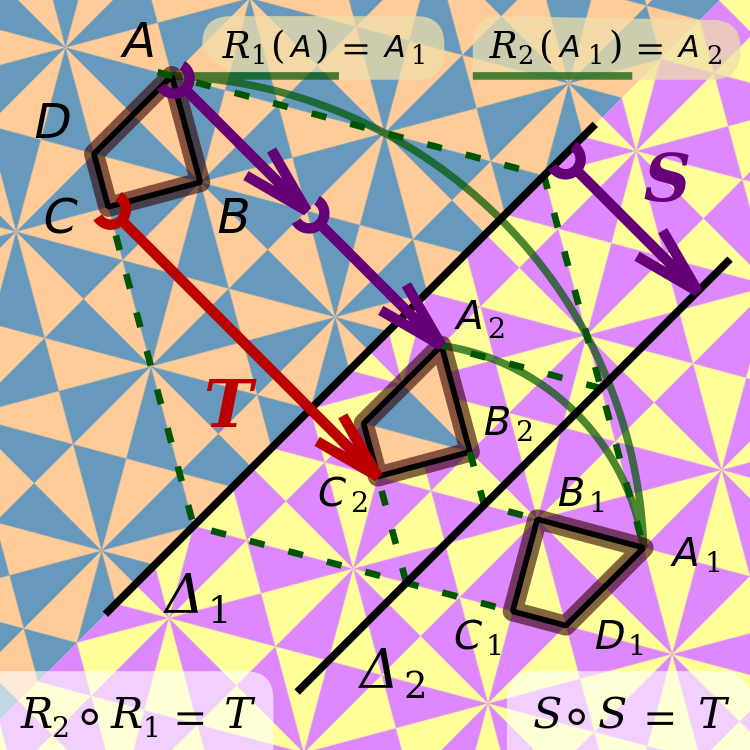
Below is a picture of Emily Benz leading a SHINE group (Goodman, 2016).
Goodman, S. (2016, February 24). Through dance, program turns “I can’t” into “I can”. Retrieved November 17, 2019, from http://news.mit.edu/2016/shine-program-teaches-math-through-dance-0224.
Another website that explores the connection between dance and math is “Dancing: Revealing the Beauty of Mathematics”. The website is written by the administration of the SEAMEO Regional Centre for QITEP in Mathematics website. The website page was published on May 26, 2017. The webpage discusses a school in Australia where students learn math through dance movements, by being creative and making their own symbols and stories for problems (Admin, 2017). Geometry is one of the easiest ways to see the relation of mathematical concepts to dance since dancers form shapes with their body, as they move their arms and legs, as well as making shapes with their formations, as groups of dancers travel around the stage.
Patterns are used in the choreography of dances as well as in the movements in relation to the beats of the music (Admin, 2017). The website is very informative about the connections between math and dance.
Admin. (2017, November 30). Dancing: Revealing the Beauty of Mathematics. Retrieved November 17, 2019, from https://www.qitepinmath.org/en/math-articles/dancing-revealing-the-beauty-of-mathematics/.
Mathematics in Dance |
Dance in Primary school encourages flexibility in thinking, creativity, and perseverance. These are all necessary for building skills for mathematics.
There is a history of Mathematics and Arts as far back as the ancient Greeks. Along with the Egyptians, they used the Golden ratio to build many of their monuments. Leonardo da Vinci incorporated mathematics as an important part of his art works.
How do dancers use Mathematics?
Different dance styles use Mathematics in different ways. For example, Latin dancers express rhythm as fractions when they talk about whole, half and quarter beats.
Ballet dancers observe the angle of the body, legs, and arms in positions such as holding a leg in the air at a 90 degree angle.
In dance we talk about our feet being in parallel and moving on diagonals. We phrase our music in counts of 8, 16 or 32.
Traditionally, in ballet, the stage is divided into quarters and eighth to position the dancers correctly for unison and for the stage lighting plan. The dance works themselves are even in two halves or three thirds.
More impressively, the dancer calculates distance between other dancers and objects throughout their performances. They even adjust these distances in response to the different size or shape of the space they perform in.
Having a heightened state of spatial awareness, ability to manipulation of space and time and proprioceptive self-imaging is a part of learning the craft of dance.
Dancers are skilled at looking for patterns in choreography and use these patterns to remember movement.
Creating a Mathematics and Dance lesson
Educationalists like Dewey, Montessori and Whitehead have identified movement as an effective way to promote learning (Evangelopoulou, 2014). Thurston (1994) believed that people were able to think on a larger scale when using spatial imagery. This was later supported by the work of Watson (2005) who investigated the ‘use of kinaesthetic experiences associated with dance.
Watson explored using dance teaching to promote engagement and learning in spatial, rhythmic, structural and symbolic aspects of mathematics’(Evangelopoulou, 2014).
The research of the late Professor Tom Cooper (Queensland University of Technology) and Dr Christopher Mathews (Griffith University) highlights that Maths and Dance is a way of bringing together the abstract and the tangible within a framework of children’s lived experiences. YuMi Deadly Maths is based on their findings and shows how the theory of bringing these two ways of learning together can work in the Primary classroom.
Starting out
Before designing your Dance and Mathematics activity it is important to watch this video to understand the phases that the lesson needs to cover to be most effective.
Erik Stern and Karl Schaffer, authors of Math Dance and founders of Dr Schaffer and Mr Stern Dance Ensemble, believe “choreographic and mathematic thinking are composed of similar building blocks”.
They involve similar processes like noting changes, remembering sequences, asking if things are bigger or smaller, checking your work to see if its consistent and much more (Stern & Scaffer, 2019 TED Talk).
How can we learn about Maths in a Primary dance class?
There are some obvious processes that lend themselves for using Maths in your dance class.
Look for a pattern.Have children identify patterns that may occur in a piece of choreography. This may be something they have learned themselves or it could be a professional piece of choreography they observe.
The pattern could be in the floor pattern (where the dancers move in the space) or in the dynamics (how the dancers move in the space) of the dance work.
Identify the pattern and represent each section with a different symbol. This teaches them about choreographic forms (eg. binary, ternary form) and about taking an abstract symbol to represent a concrete idea.
Guess and CheckThe children approximate how far each movement travels across the floor and then draw a model of their dance including the actual measurements of each movement.
Reduce the size of the space and repeat the process.
They then create a table that answers the following questions. What is the difference between the movements in the first model and the movements in the second? How did you have to change the movements to adapt it to the second version?
Ensure that a part of the table allows them to show their working.
These movement activities require advanced thinking skills in both mathematics and dance.
Try to use Polya’s Problem Solving Model when doing these kinds of activities by dividing the lesson into Think, Plan, Do and Lookback activities. It fits nicely with a creative Arts approach and encourages children to see further synergies in the processes used for problem solving in Dance and Mathematics.
Themes and ideas for teaching Mathematics Dance
The obvious themes that link Mathematics to Dance are about shapes, patterns, angles, symmetry, geometry, multiplication, subtraction, division, and addition. However, by looking at the dancers’ bodies in relation to one another, and the space in which the dancer moves, we can design activities that require more complex consideration.
Patterns of beats and rhythmic changes in the music or the way the body moves and responds to the music can also be a starting point for ideas.
Shape transformation that investigates reflection, rotation and translation are also great for dance activities.
Look at the statistical properties of a piece of dance. How many jumping movements were there versus balancing ones? Sharp versus sustained? Suspended versus falling?
Social dances are wonderful ways to investigate Maths as they are about symmetry, changing patterns and are easy for children to illustrate from an overhead view. The Maypole dance offers many challenges for the Maths students as you can look at the size of the pole, the angle of the ribbons (, the distance between the dancers and the maypole and the percentage that it diminishes through the course of the dance.
For an older age group, you could design an activity where they predict how many geometric patterns using 6 people with different coloured ribbon combinations are created. But perhaps we are now looking at proofs!
You can see how exciting Mathematics and Dance can be.
Using dance to teach about mathematics and mathematics to enhance learning about dance can result in positive learning experiences for Primary students.
Using dance in your Maths classroom can lead to a better understanding of mathematical concepts, increase motivation, and encouraged creativity and critical thinking. It acts to acknowledge the part number and logic plays in the art of Dance in a professional environment and makes students see the real-world applications of Maths learning in a creative context.
For more ideas about how to integrate Dance in your Maths class see Dance and Maths: Angles and the free lesson plan Coding for Kids.
References
Evangelopoulou, Polyxeni (2014). The impact of integrating dance and movement in maths teaching and learning in preschool and primary school settings. Masters research (International and Comparative Education). Stockholm University.
Thurston, W. P. (1994). On proof and progress in mathematics. Bulletin of the American Mathematical Society, 30 (2), 161-177.
Watson, A. (2005).
Dance and Mathematics: Engaging Senses in Learning. Australian Senior Mathematics Journal, 19 (1), 16-23.
MATHEMATICS IN CHOREOGRAPHY
- Authors
- Executives
- Job files
- Award documents
Kurapova E.S. 1Maidanova E.D. 1
1
Buldakova G.M. 1
1
The author of the work was awarded a diploma of the winner of the II degree
Diploma of a school student Certificate of the head
The text of the work is placed without images and formulas.
The full version of the work is available in the "Files of the work" tab in PDF format
Introduction
Where there is beauty, the laws of mathematics work there.
G. H. Hardy
The two main principles in human culture are science and art, two complementary forms of human creative activity.
From birth, we are surrounded by the world of precise calculations. We are so close to mathematics that we simply do not notice it. The original meaning of the word "mathematics" (from the Greek mathema - knowledge, science, in particular, in Latin ars mathematica, means the art of mathematics) has not been lost even today, it remains a symbol of wisdom, the queen of all sciences. The beauty of mathematics among the sciences is very great, and beauty is one of the links between science and art. Of the many arts that mathematics interacts with, we chose choreography.
Since the most ancient centuries, there has been dance in human life. Among primitive people, love, work and ritual are embodied in dance movements. Each of us at least once danced or watched the performance of the dance. What a mesmerizing sight it is! It is often said: "Dance is the secret language of the soul." Has anyone wondered how much mathematics is in this secret language?
Based on many years of experience in choreography, we know that dance has much in common with such a science as mathematics, since rhythm, tempo, dance figures obey certain mathematical laws and concepts.
Actuality:
Mathematics is inextricably linked with everyday life, in which there is a place for dancing. Mathematical tasks develop thinking, logic, a set of analytical skills: the ability to group objects, reveal patterns, determine relationships between phenomena, make decisions.
It has also been proven that dancing has a beneficial effect not only on the physical form, but also on mental abilities, spatial imagination. Thus, a reasonable combination of dancing and mathematics allows you to develop mental abilities.
Hypothesis: many dance movements are easier to perform if you know their mathematical component.
Purpose of study :
Find common ground between mathematics and choreography by studying dances based on the construction of geometric figures and dance movements from the point of view of mathematical accuracy.
To achieve this goal, we put forward the following tasks :
1. Study the literature on the research topic;
2. Compare and contrast common terms and concepts in mathematics and choreography;
3. Analyze the results;
4. Show the application of the acquired knowledge in choreography;
5. Promote dance classes as a guarantee of a healthy lifestyle.
Object of research: mathematics and dance.
Subject of study: the mathematical component of the dance.
In the process of research, we used the following methods : study, comparison, comparison, analysis.
The novelty of the study lies in the fact that behind the dance plasticity we managed to see not only the creation of dance figures, but also the exact mathematical calculation.
I. Dance pattern as one of the expressive means of choreography
To date, there are not as many interpretations of the concept of "dance pattern" in modern literature as we would like. Famous ballet dancer and choreographer Zakharov R.V. gives us a definition of the concept of “dance pattern”, which in choreography has a double meaning: “drawing is the movement of dancers across the stage and that imaginary trace that, as it were, remains on the floor, fixing possible dance figures and forms of movement around the stage.”
The centuries-old experience of ballet masters of the past years allows modern choreographers to use the available information and enrich their dance compositions with various means of choreography: new vocabulary (movements and tricks), options for rearrangements in dance or dance pattern (all types of dance pattern), lighting design, pantomime and artistic game of the dancer, musical accompaniment with costumes and scenery.
Having looked at various productions by modern choreographers, one can see that the pattern of the dance remains the same, only the variations of their application in modern choreography change. Modern dance pays more attention to the graphics of movement, rhythm and angle of performance.
II. Dance Algebra
2. 1. Mathematics in music
It is hard to imagine dance without music, which, as it turned out, is closely related to mathematics. We will name the most significant points of contact.
In music, note durations are used, the names of which are also the names of ordinary fractions. For example, a whole is 4/4, a half is 1/2, a quarter is 1/4, an eighth is 1/8. The beats in music add up (eighth + eighth = quarter), just like fractions in mathematics (1/8 + 1/8 = 1/4).
The change in the pitch of the scale is a geometric progression.
The alternation of strong and weak beats determines the musical size (m / r) of the work, on which the form of music (type of musical composition) depends. The choreography uses music from m/r 2/4 (polka), 3/4 (waltz), 4/4 (march). All movements in the dance must correspond to the music ("submit" to the beat) - highlight the strong beats and smoothly "work out" the weak ones. For example, in rumba, the step falls on the beat itself (the “one” count is a strong beat), and the duration of the note (the “and” count is a weak beat) is the work of the hips. The longer the “and” count, the faster and more expressive the rumba.
Each musical genre has a certain rhythmic pattern and tempo, the totality of which sets the choreography of the dance. Fast tempos are inherent in music that embodies rapid movement, cheerfulness (for example, the image of Odile from the ballet "Swan Lake"). Slow tempos are characteristic of music that reflects a state of calm, deep sadness (for example, the image of Odette from the ballet "Swan Lake").
Thus, the music determines the nature of the dancer's movement, organizes his movements and gives an emotional coloring to the production.
2.2. Algorithm
Since mathematical science is associated with the concept of an algorithm (“step by step”) and sequence, and a dance step is both a sequence and an order of movements, it follows that dance and mathematics are connected by a common concept - “step”.
Algorithms are used to perform tasks of the same type in order to speed up the process of achieving results. The essence of the algorithm is that, having learned to perform elementary operations, in the future we do not think about the order in which they are performed.
As in mathematics, dance has its own set of aspects that must be mastered in the early years of training in order to bring them to automatism.
Due to the variety of dance vocabulary, compositions, figures and poses, modern choreography manuals present an algorithm for teaching classical dances.
2.3. Account
Score is an important concept, without which it is difficult to disassemble the dance into its component parts. To calculate music, you need to hear the rhythm. The easiest way to do this is by listening to percussion instruments. There are more precise ways to break music into squares (four counts) and eights. But in order to dance "in time" it is not necessary to receive an academic musical education, it is enough to follow the rhythm.
We see the dance, as the reader sees the work, penetrating into its meaning, but not always thinking about what is behind the art. But the birth of beauty is always a complex, time-consuming process, involving the limiting capabilities of a person.
The same can be said about ballroom dancing: external weightlessness is not easy, and sometimes the only way for dancers to the stars lies through those thorns. For example, if we talk about cha-cha-cha, then the assessment here takes into account such indicators as placing a foot on a strike at the expense of 1,2,3,4, and the time it takes to complete a step, measured in fractions seconds, etc. In every dance, counting is important, and we learn to count only with the help of mathematics. When you hear music, you must time your movements correctly to get into the rhythm. This is where math comes in handy, correct counting will improve your understanding of the dance, and you will stop straying when you understand the dance count.
| Dance name | Time signature | Temp | Account |
| Slow waltz | 3/4 | slow | 1, 2, 3 |
| Tango | 2/4 | moderately fast to fast | 12 |
| Quickstep | 4/4 | 200 beats per minute, fast | 1, 2, 3, 4 |
| Slow Foxtrot | 4/4 | 120 beats per minute, truly slow in natural calmness | 1, 2, 3, 4 |
| viennese waltz | 3/4 | 80 beats per minute, fast. | 1, 2, 3 |
| paso doble | 2/4 | quick | 12 |
| Samba | 2/4 | 60 strokes per minute, fast | 12 |
| Cha-cha-cha | 4/4 | 120 beats per minute, moderately fast | 1, 2, 3, 4 |
| Rumba | 4/4 | slow | 1, 2, 3, 4 Fast", "Fast" and "Slow" |
| Jive | 2/4 | fast, very fast | 12 ; 3&4, 3&4 |
2.
4. Function Graphs
It is impossible to create a beautiful dance without graphs of mathematical functions. A beautiful dance is a beautiful schedule. If you analyze the basic ballet position, when the arms are above the head, you will notice that two curves are laid in it - one on the right, the other on the left. The use of the epaulement ballet position, which is the highest achievement of the great ballet dancers, generates a huge number of counter-rotations, determined by the relationship between the legs, arms, head and even eyes. In each dance movement, you can find a graph of one of the mathematical functions
| Formula | Function Graph | dance movement |
| y = x | ||
| y = x 2 |
III.
Dance Geometry
Movement is a change in the plane, in which the size and shape of objects are preserved. Examples of movements are symmetry, rotation, and parallel translation. Such geometric movements can be found in many dance performances, especially if the dance is built on the synchronous execution of movements.
Symmetry is a fundamental property of nature. In ancient times, the word "symmetry" was used in the meaning of "harmony", "beauty".
3.1. Symmetry and asymmetry
1. Symmetry in mathematics
Let us turn to the concepts of symmetry and asymmetry. In mathematics, the following types of symmetry are distinguished: central, axial, mirror.
Central symmetry is a mapping of space onto itself, in which any point A passes into a point A 1 symmetrical to it relative to the given center.
In the figure - symmetry, about a point.
Axial symmetry - mapping of space onto itself, in which any point A passes into a point A 1 symmetrical to it with respect to the given axis.
Mirror symmetry
Mapping of space onto itself, at which any point P goes into a point P 1 symmetrical to it with respect to this plane .
Asymmetry - absence or violation of symmetry.
2. Symmetry in dance
Symmetry is a harmonious, easy-to-observe element of choreography that is necessary to create the basic structure of the dance.
Symmetry in dance is a calm, unruffled, logical and simple element of choreography. The principle of symmetry can be traced in many early ballets, where dancers lined up in equal numbers and formed a homogeneous structure on the stage, having a center and (or) axis of symmetry.
Symmetry is also called the situation in which all dancers perform the same movement at the same time. Symmetry is the balanced arrangement of the dancer's body, the location of the dancer's body in space. For example, a ballerina ends her number with a sparkling fouette when she spins on one leg a certain number of times. It is the repetition of these movements (the leg repeatedly describes a circle that has many axes of symmetry - diameters and a center of symmetry) that determines their aesthetic effect.
In choreography, several types of symmetry are distinguished;
| 1) Symmetry of ballet positions of legs, arms, body, head | |
| 2) Symmetry of the dance pattern (the formation of a homogeneous structure on the stage in lines and drawings - a circle, a wedge, a square, etc. | |
| 3) Symmetry of executed movements |
) However, to produce the desired effect, symmetry must be accompanied by asymmetry.
Asymmetry is an unexpected and unusual element, and therefore makes the dance more interesting for the observer. It reveals the movements to a greater extent, makes the dance alive, saturating it with unpredictable elements. This principle is more commonly used. An example is the image of a jester from the ballet "Swan Lake".
Thus, using the principles of symmetry and asymmetry, the choreographer achieves the exact expression of his idea. The key to creating a successful production is the harmony between the two principles.
3. 2. Parallelism
1. Parallelism in mathematics
Consider the concept of parallelism.
In geometry, there are several types of parallelism: parallelism of lines, parallelism of a straight line and a plane, parallelism of planes.
Parallelism of lines on a plane
Two lines in a plane are said to be parallel if they do not intersect AB⃓CD
Parallelism of lines in space
Two lines in space are called parallel if they lie in the same plane and do not intersect. a , b , a b
Parallelism of line and plane
A line and a plane are called parallel if they have no common points.
a, a b, b.
Parallelism
Two planes are said to be parallel if they do not intersect.
2. Parallelism in dance
| 1) Position parallelism The execution of positions is the beginning of the beginnings of learning choreography. The feet of dancers in standard dances must be parallel to each other. There are five foot positions in classical dance. | |
| 2) Parallelism of partners When dancers perform the same elements of the dance, synchronization must be observed. The coordination of movements of one partner to another is characterized by the parallelism of each part of the body of one dancer to another. | |
| 3) Parallelism to the floor In dance, there are certain standards for the correct execution of movements. |
One of the standards is the parallelism of body parts to the floor when performing a dance element. So, for example, grand jet 9 - a jump in which both legs of the dancer must be parallel to the floor, etc. It follows from the above that parallelism is necessary to coordinate movements in time and space, improve the technique of performance, as well as the eversion of the legs during the dance.
3.3. Perpendicularity
1. Perpendicularity in mathematics
In geometry, there are: perpendicularity of lines, perpendicularity of a straight line to a plane and perpendicularity of planes.
Perpendicularity of straight lines on a plane
Two intersecting lines are called mutually perpendicular if they form four right angles.
a b
Perpendicularity of lines in space
Two lines in space are called mutually perpendicular if the angle between them is 90˚.
Straight plane perpendicularity
A line is said to be perpendicular to a plane if it is perpendicular to any line in that plane.
Plane perpendicularity
Two intersecting planes are said to be mutually perpendicular if the angle between them is 90˚. ⊥
2. Perpendicularity in dance
In dance, the following types of perpendicularity are distinguished:
| 1) Perpendicular to the floor An element of dance in which any part of the body is perpendicular to the floor. | |
| 2) Perpendicularity of body parts An element of dance in which body parts are perpendicular to each other (for example, grand batman 8 ). |
Consequently, the perpendicularity in the dance gives expressiveness, enchantment, expression to the dance, and is also not only an indicator of the dancer's professionalism.
3.4. Turn
1. Turn in mathematics
It is said that point A 1 of the plane is obtained from point A by turning around the point O by an angle if OA 1 = OA and AOA 1 = . A transformation of the plane, in which a given point O remains in place, and all other points rotate around the point O in the same direction (counterclockwise or clockwise) by a given angle, is called a rotation around the point O by an angle.
2. Dance turn
Turn - this term means a turn around its axis by 360° - (one turn), a half turn - a turn by 180°, a quarter - by 90°, two turns - 720°, etc. If the execution of the turn does not fit into the account, but takes, let's say, two accounts, we start the turn on the first account, and on the second - we twist. If the movement starts on one count and ends on the next, the movement is completed.
Fuete is a series of consecutive rotations in place, during which the leg opens 45 degrees with each rotation.
There is a moment in Swan Lake where you need to perform exactly 32 fouettes (the very end of the pas de deux of Odile and the Prince, the maximum number of fouettes is 32 pieces, according to the number of musical measures). The first Russian ballerina to show her 32 fouettes to the most respectable audience was the main star of the Mariinsky Theater, Matilda Kshesinskaya.
3.5. Angle
1. Angle in mathematics
Angle is a geometric figure that consists of a point and two rays emanating from this point. These rays are called the sides of the angle, and their common origin is called the vertex of the angle. The unit of measure for angles is degrees.
In mathematics, the following types of angles on a plane are distinguished depending on the degree measure of the angle:
| Straight - angle equal to 90 ° | Spicy - angle less than 90 ° | Stupid - angle over 90 ° | Expanded - angle equal to 180 ° |
2.
Dance Angle
Degree measures are directly related to ballet. Many movements associated with lifting the leg are measured in degrees. Of course, the ballerina should not raise her leg by the exact number of degrees, they are spoken about approximately, so that the ballerinas have an idea - in what movements how much the leg rises.
The following types of angles are represented in the dance:
| Batman tandu 5 30 ° | Batman Jete 6 45 ° | Batman devlope 4 90 ° | Arabesque 3 120 ° |
A la zgond - a pose in which the leg through the 2nd position is raised to the side above 90 degrees.
Tour - rotation of the body around the vertical axis by 360 degrees.
Ambuate - jump with alternately throwing forward or backward legs bent at the knees by 45 ° or 90 °.
Pirouette - 360 degree turn on one leg
Assemble (assemble, French - assembled), in classical dance, a jump with throwing the leg forward, sideways and back at an angle of 45 ° (petit A.) and 90° (grand A.)
3.6. Point
The dancers during the performance take various poses, alternating with other elements of the dance.
A posture in dance is understood as a stop in motion, in which the dancer's body is in a stationary position of balance. In choreography, this is called a "dot".
Also, the concept of "point" is used when performing any turn or rotation, when it is important to maintain balance. This is obtained precisely due to the ability to focus the gaze or “keep the point”.
3.7. Direct (line)
An important mathematical concept is a straight line. In mathematics, we study such lines as a straight line, a parabola, a hyperbola, a sinusoid.
In ballet schools, dancers are taught to work with lines in space, since every dance pattern is made up of lines (straight lines). During the dance, when one pattern replaces another, the dancer must "keep the line", that is, adhere to the trajectory along which the rebuilding occurs. One of the main criteria for evaluating a dance is beautifully and correctly lined lines.
3.8 Circle
1. Circle in mathematics
There are two separate concepts: circle and circumference.
A circle is a geometric figure consisting of all points located at a given distance (circle radius) from a given point (circle center).
The circle is the part of the plane bounded by the circle.
2. Circle in dance
In choreography, the circle is predominantly seen in the dance pattern. In their own work, choreographers and choreographers often use a different name - a circle.
The oldest type of Russian folk dances, in particular northern , is a round dance that forms a circular structure. Often you can find a double circle (a circle within a circle).
Sometimes the dancers form two circles side by side, and sometimes these circles seem to overflow one into the other and their movement forms a figure-eight pattern. Large and small circles are a very common form of building a Russian round dance.
The circle is viewed:
| 1) In the dance pattern | 2) In hand positions |
| round dance - circle in a circle | preparatory position 3rd position |
| 3) In the classic exercise 10 | 4) In motion |
Thus, it is impossible to imagine a dance pattern without basic geometric concepts: a point, a line, an angle.
The aesthetics of geometric form, in particular the aesthetics of the line, attracted the attention of not only mathematicians. The dance of any ensemble is based on the construction of dance figures. The simplest beautiful figure is a circle; she makes a good impression on us.
3.9 Geometric figures
1. Figure in dance - a position, a position taken by someone when performing something in motion; part of the dance; in ballroom dance and ballet - a combination of several dance steps (pas), interconnected and arranged for a certain number of measures of music.
Various geometric shapes are built in the dance. The pattern of the dance is the location and movement of the dancers on the stage.
| Dance | figure in dance | Geometric figure |
| round dance | "Wall" | Parallel lines |
| "Wheel" | Circle | |
| "Double Circle" | concentric circles | |
| "Wedge" | Two rays with a common vertex that form a non-flattened angle. | |
| "Star" | Diagonals of a square intersecting in the center | |
| "Eight" | Two circles with external touch | |
| Dance | figure in dance | Geometric figure, line. |
| Waltz | Waltzing in a straight line | Polyline with equal links and angle of inclination of adjacent links |
| A circle | Circle | |
| Samba | Cross | Diagonals of a square or rhombus that intersect at the center |
| Cha-cha-cha, rumba | Fan | circle sector |
| Track | Polyline with periodically repeating segments | |
| Viennese, quickstep | A circle | sinusoid |
2.
Equilibrium and balance
Each dance figure can be mentally inscribed in an n-gon. A geometric figure is stable if the center of gravity is correctly calculated. The center of gravity is the point through which the line of action of the resultant elementary forces of gravity passes. The center of gravity of the human body does not have a constant anatomical location within the body, but moves depending on changes in posture; its excursions relative to the spine can reach 20-25 cm .
The stable figures include a square, a rectangle, a triangle with a larger side at the base, a trapezoid with a large lower base.
The dancers take a stable pose on a strong musical beat, with a long performance, at the end of the dance composition.
The unstable figures include a rhombus, a triangle with a smaller side at the base, a triangle based on the top, a parallelogram, a circle.
Unstable postures are characterized by instantaneous execution of the element, acrobatic character. The dancer takes them at a high musical intensity. Unstable elements, in addition to postures, include jumps, runs, turns.
So, balance (stability) is of particular importance for dance. Balance is affected by the projection of the common center of gravity. The closer to the support the center of gravity of the body and the larger its area, the higher the stability of the body.
Conclusion
As part of the study, the mathematical component of the dance was revealed. The dance contains figures, fractions, proportions. Another fact confirming the connection between dance and mathematics is the use of common terms: lines, diagonals, in the dance pattern can be parallel or perpendicular, symmetrical or asymmetrical. In addition to visible geometric shapes and algebraic forms, the dancer always has a sense of balance, center, that is, the dancer is in the coordinate system.
Behind the dance plasticity one can see not only the creation of poses, geometric figures, drawings, but also the exact mathematical calculation of the strength of the jump, the number of turns in the round, the length and width of the step. Since mathematical science is associated with the concept of an algorithm (“step by step”), and sequence, it turns out that dance and mathematics are connected by a common attribute - “step”. A dance step is both a sequence and an order of movements. The mathematical component of the dance is not only visible, but also felt.
It is impossible to measure the beauty and harmony of dance with geometry alone. At the same time, it is geometry that helps dancers find new perfect figures and diversify the pattern of the dance.
A mathematician, like an artist or a poet, creates patterns and his patterns must be beautiful, ideas, just like words or colors, must harmonize with each other. Beauty is the first requirement: there is no place in the world for ugly mathematics, just as there is no place for ugly dance.
If there is such an opportunity, then mathematicians need to draw more, dance more, play more music - in this case, new invisible aesthetic horizons of mathematical creativity will open up for them.
Our hypothesis that many dance movements are easier to perform, knowing their mathematical component, was confirmed. We began to take a different attitude to those dance movements that we study under the guidance of our choreographer Lyubov Pavlovna Karpukhina.
In conclusion, we would like to add that for the part of the youth that has not yet chosen what kind of sport to do, we suggest paying attention to dancing. This is a healthy lifestyle, and beauty, and an accurate mathematical calculation.
And also, according to physiologists from Harvard University (USA), schoolchildren who have completed a one-year dance course pass geometry tests better than those who have never danced or received only a few dance lessons.
A Canadian study found that professional dancers performed better on attention tests than amateur or non-dancers.
We have been in the Expression dance group for 7 years. We study well at school, we manage to engage in social activities.
It's never too late to start dancing. A multi-year study conducted at the Einstein College of Medicine in New York showed that older people who dance regularly are 76% less likely to fall into senile dementia compared to those who never dance. Moreover, regular reading is effective in preventing dementia by only 35%, solving crossword puzzles by 47%, and playing sports does not help at all.
It is known that many dance steps have a beneficial effect on the human nervous system: they relieve nervous tension and headaches, stabilize blood pressure; in dancers, the amount of oxygen in the blood increases by 18%; dancing burns about 400 kcal per hour; attending a dance club at least twice a week reduces the risk of dementia; professional dancers don't get Parkinson's disease.
Bibliography
1. Atanasyan L.S., Butuzov V.F., Kadomtsev V.F. and etc. Geometry. Textbook for grades 10-11 of educational institutions. M.: Education, 2014.
2. Atanasyan L.S., Butuzov V.F., Kadomtsev V.F. and etc. Geometry. Textbook for grades 7-9 of educational institutions. M.: Education, 2013.
3. Azevich A. I. Twenty lessons of harmony: Humanitarian and mathematical course. - M .: School-Press, 1998. - 160s.: ill. (Library of the journal "Mathematics at School". Issue 7).
4. Voloshinov A.V. "Mathematics and Art" - M .: "Enlightenment" 2000
5. Children's encyclopedia. Art. For middle and older age. - 3rd ed. - M .: "Pedagogy", 1977. -576 p.
6. L. F. Pichurin, Behind the pages of a textbook of algebra: kn. for students in grades 7–9 avg. school - M .: Education, 1990. - 224 p.: ill.
7. Internet resources:
http://ru.
wikipedia.org/wiki/
http://danceeurope.narod.ru- the art of dance
http://www.fiziolive.ru - ballroom dancing
http://new.ug.ru/archive - and there is a mathematical calculation in the dance
http://images.yandex.ru - pictures
http://edugalaxy.intel.ru – choreography of mathematics
http://nedug.ru - salvation from diseases - in dance
http://studope dia.ru - types of folk dance
http://kindlebjjk.ru - mathematics and ballet
http://cyberleninka.ru- mathematics of ballet
25
Work views: 11767
Mathematics in dance
- Authors
- Executives
- Job files
- Award documents
Gedygusheva A.M. 1
1GBOU of Moscow "School No.
354 named after D.M. Karbyshev"
Postavnichiy Yu.S. 1
1SBEI of Moscow "School No. 354 named after D.M. Karbyshev"
The author of the work was awarded a diploma of the winner of the II degree
Diploma of a school studentCertificate of the head
The text of the work is placed without images and formulas.
The full version of the work is available in the "Files of the work" tab in PDF format
Introduction
Science and art are two main principles in culture, two complementary forms of human creative activity.
From birth, we are surrounded by the world of precise calculations. The original meaning of the word "mathematics" (from the Greek mathema - knowledge, science, in particular, in Latin ars mathematica, means the art of mathematics) has not been lost even today, it remains a symbol of wisdom, the queen of all sciences.
The beauty of mathematics among the sciences is very great, and beauty is one of the links between science and art. Of the many arts with which mathematics interacts, I decided to choose choreography.
Since the most ancient centuries, there has been a dance in human life. Among primitive people, love, work and ritual are embodied in dance movements. Each of us at least once danced or watched the performance of the dance. Just think how much mathematics is in the dance!
Dance contains figures, fractions, proportions. Another fact that confirms the connection between dance and mathematics is the use of common terms: lines, diagonals, in a dance pattern can be parallel or perpendicular, symmetrical or asymmetrical. The paper presents graphical implementations of the sections described in the paper.
In addition to visible geometric figures and algebraic forms, the dancer always has a sense of balance, center, that is, the dancer is in the coordinate system.
Behind the dance plasticity one can see not only the creation of poses, geometric figures, drawings, but also the exact mathematical calculation of the strength of the jump, the number of turns in the round, the length and width of the step. Many dance moves are easier to perform if you know their math.
Dance is both a healthy lifestyle, and beauty, and an accurate mathematical calculation.
Problem: Knowing math makes it easier to learn dance.
Relevance: My many years of experience in choreography shows that dance has much in common with such a science as mathematics, since rhythm, tempo, dance figures obey certain mathematical laws and concepts.
Mathematics is inextricably linked with everyday life, in which there is a place for dancing. Mathematical tasks develop thinking, logic, a set of analytical skills: the ability to group objects, reveal patterns, determine relationships between phenomena, make decisions.
Dancing has a beneficial effect not only on the physical form, but also on mental abilities, spatial imagination. A reasonable combination of dancing and mathematics allows you to develop mental abilities.
The purpose of the work: to identify points of contact between mathematics and choreography on the example of studying dances based on the construction of geometric figures and dance movements in terms of mathematical accuracy
Tasks:
1. study dance movements and associate them with mathematical concepts;
2. analyze the connection between dance and mathematics;
3. consider applying the acquired knowledge in the choreography.
Research object: Choreographic movements.
Subject of study: Mathematical description of choreographic movement.
Hypothesis: dance and mathematics are closely related.
Research methods:
1. Working with educational and popular science literature, Internet resources;
2. Observation, comparison, analogy;
3. Analysis of own practical experience.
1. Basic concepts
Dance – an art form in which an artistic image is created through rhythmic plastic movements and a change in the expressive positions of the human body.
Algorithm
In everyday life, each person is faced with the need to solve problems of varying complexity. Some of them are difficult and require long reflections to find solutions, while others, on the contrary, are so simple and familiar that they are solved automatically. At the same time, the execution of even the simplest task is carried out in several successive stages (steps). In the form of a sequence of steps, it is possible to describe the process of solving many problems known from the school mathematics course. Since mathematical science is associated with the concept of an algorithm (“step by step”) and sequence, and a dance step is both a sequence and an order of movements, it follows that dance and mathematics are connected by the general concept of “step”.
Algorithms are used to perform the same type of tasks in order to speed up the process of achieving the result. The essence of the algorithm is that, having learned to perform elementary operations, in the future we do not think about the order in which they are performed. As in mathematics, dance has its own set of aspects that must be mastered in the early years of training in order to bring them to automatism.
Dance can be considered not only as a sequence of interrelated movements, but also as an algorithm.
American choreographer William Forsythe created a dance technique he called "Dance Geometry" or "Technology of Improvisation". Using this technique, the dancer draws imaginary geometric shapes in the air and then drags their limbs through this complex and invisible geometry. It turned out a new interesting direction in modern dance.
In the modern world, a dance has appeared, called "Technology of Improvisation". Using this technique, the dancer draws imaginary figures in the air and then drags his limbs through this complex and invisible geometry.
To make this geometry visible, a computer animation superimposed on a motion video is used. First, the dancers imagine lines in space that could curve, ripple, or deform in other ways.
Moving from a point to a line, then to a plane, then to a volume, they visualize a geometric space made up of individual points with a huge number of interconnections. Since these points are inside the dancer's body, no relative movement is required.
2. Relationship between dance and mathematics
2.1 Function curves
It is impossible to create a beautiful dance without graphs of mathematical functions. A beautiful dance is a beautiful schedule. In each dance movement, you can find a graph of one of the mathematical functions.
Often, when dancers spread their arms or even legs in different directions, it turns out - a straight line. And when they stand in a circle, it turns out a circle.
If you stand in attitude, this is one of the basic postures in classical dance, in which the balance is maintained on one leg, and the other leg is raised and laid back in a bent position, you get a broken line.
If you take the third open position of the hands in a classical dance, you get a parabola. If we cross pairs of partners' hands and make wave movements, we will involuntarily get the sign of infinity.
2.2. Geometric shapes
Angle is a geometric figure that consists of a point and two rays emanating from this point. These rays are called the sides of the angle, and their common origin is called the vertex of the angle. The unit of measure for angles is degrees. In mathematics, the following types of angles on a plane are distinguished depending on the degree measure of the angle: acute, straight, obtuse and developed.
Vertical twine
During the performance, the dancers take various poses, alternating with other elements of the dance. A posture in dance is understood as a stop in motion, in which the dancer's body is in a stationary position of balance. In the choreography it's called " dot ".
Also, the concept of "point" is used when performing any turn or rotation when it is important to maintain balance. This is obtained precisely due to the ability to focus the gaze or “keep the point”.
Point - body position. In order for a continuous and sometimes quite fast movement to be learned at a slow pace, the dance is considered as a series of postures connected by intermediate movements, each of which is called a point. If a stop occurs at one of the points, it is called a fixed point. If there is no stop or it is taken conditionally in order to understand through what intermediate stages the movement from one fixed point to another occurs, we speak of the presence of a passing point, that is, the position of the body in which we do not linger.
An important mathematical concept is straight line . In ballet schools, dancers are taught to work with lines in space, since every dance pattern is made up of lines (straight lines). During the dance, when one pattern replaces another, the dancer must "keep the line", that is, adhere to the trajectory along which the rebuilding occurs. One of the main criteria for evaluating a dance is beautifully and correctly lined lines.
In hand positions
(3rd position)
In mathematics, two separate concepts are distinguished: circle and circle . In choreography, the circle is predominantly seen in the dance pattern. In their own work, choreographers and choreographers often use a different name - a circle.
On the move
The most ancient form of Russian folk dances is round dance, which forms a circular structure. Often you can find a double circle (a circle within a circle). Sometimes the dancers form two circles side by side, and sometimes these circles seem to overflow one into the other and their movement forms a figure-eight pattern. Large and small circles are a very common form of building a Russian round dance.
2.3. Symmetry and asymmetry
In mathematics, there are several types of symmetry: axial (relative to a straight line), central (relative to a point), mirror (relative to a plane). Symmetry in dance is a harmonious, easy-to-observe element of choreography that is necessary to create the basic structure of the dance. There are also several types of symmetry in the dance: the symmetry of the ballet positions of the legs, arms, body, head; symmetry of the dance pattern; symmetry of the movements performed.
Symmetry in dance is a calm, unruffled, logical and simple element of choreography. The principle of symmetry can be traced in many early ballets, where dancers lined up in equal numbers and formed a homogeneous structure on the stage, having a center and (or) axis of symmetry. Symmetry is also called the situation in which all dancers perform the same movement at the same time. Symmetry is the balanced arrangement of the dancer's body, the location of the dancer's body in space. For example, a ballerina ends her number with a sparkling fouette when she spins on one leg a certain number of times.
It is the repetition of these movements (the stem repeatedly describes a circle that has many axes of symmetry - diameters and a center of symmetry) that determines their aesthetic effect.
Matilda Kshesinskaya became the first Russian ballerina to perform 32 fouettes at the Mariinsky Theatre.
Symmetry of ballet positions of legs, arms, body, head
Dance Symmetry
(formation of a homogeneous structure on the stage in lines and drawings)
However, to produce the desired effect, symmetry must be accompanied by asymmetry.
Asymmetry is an unexpected and unusual element, and therefore makes the dance more interesting for the observer. It reveals the movements to a greater extent, makes the dance alive, saturating it with unpredictable elements. This principle is more often used than symmetry.
Thus, using the principles of symmetry and asymmetry, the choreographer achieves the exact expression of his idea. The key to creating a successful production is the harmony between the two principles.
2.4. Parallelism and perpendicularity
As in mathematics, in dancing it is necessary to observe a clear construction of figures. In order for the viewer to see beautiful choreography on stage, parallelism is used: parallel positions, parallel to a partner, parallel to the floor.
When dancers perform the same elements of the dance, synchronization must be observed. The coordination of movements of one partner to another is characterized by the parallelism of each part of the body of one dancer to another. This principle in dance is called the "Principle of Parallel Partners".
Also in the dance there are certain standards for the correct execution of movements. One of the standards is the parallelism of body parts to the floor when performing a dance element. So, for example, a grand jet is a jump in which both legs of the dancer must be parallel to the floor, etc.
Perpendicularity is used in the same way. Perpendicular to the floor - an element of dance in which any part of the body is perpendicular to the floor. Perpendicularity of body parts - an element of dance in which body parts are perpendicular to each other (for example, grand batman).
2.5. Turn
Turn - this term means a turn around its axis by 360 ° (one turn), half a turn - a turn by 180 °, a quarter - by 90 °, two turns - 720 °, etc.
Fuete is a series of consecutive rotations on the spot, during which the leg opens 45 ° with each rotation.
Tour - rotation of the body around the vertical axis by 360°. Pirouette is a 360° turn on one leg.
Each dance figure can be mentally inscribed in an n-gon. A geometric figure is stable if the center of gravity is correctly calculated. The center of gravity is the point through which the line of action of the resultant elementary forces of gravity passes. Stable figures: a triangle with a larger side at the base, a trapezoid with a large base at the bottom, a square, a rectangle.
Conclusion.
In my work I compared dance with mathematics. Based on the foregoing, we can conclude that the dance pattern contains geometric shapes, lines, diagonals, circles. Dancers move along their trajectories, subordinating their movements to the musical rhythm and tempo. They can be parallel or perpendicular, symmetrical or asymmetrical. Moreover, the position of the body in the dance is fixed by various angles - sharp, obtuse, straight.
In addition to visible geometric shapes and algebraic forms, the dancer always has a sense of balance, center, that is, the dancer is always in the coordinate system.
Behind the dance plastique we can see not only the creation of poses, geometric figures, drawing, but also the calculation of the strength of the jump, the number of turns in the round, the length and width of the step, the acceleration and deceleration of movement. Thus, mathematical logic is manifested in the dance, the laws of algebra and geometry are used.
Therefore, using the algorithm for teaching classical dances, basic mathematical concepts and principles, the choreographer achieves an accurate expression of his idea, which is the key to creating a beautiful and successful dance production.
Linking the elements of the dance, we build logical chains. Spatial imagination develops.
Dancing is a good way to train your intellect! Schoolchildren who have completed a one-year dance course do better on tests in geometry and mathematics than those who have never danced (Harvard University, USA). Professional dancers perform better on attention tests (Studies in Canada).
So, the mathematical component of the dance is contained in the pattern of the dance, in the movements of the dancer, in the classical positions. Dance is both a healthy lifestyle, and beauty, and an accurate mathematical calculation.
Literature:
Basics of classical dance. Edition 6. Series “Textbooks for universities. Special literature" - St. Petersburg: Publishing house "Lan", 2000.
Okunev. M. Russian folk round dances and dances: a methodological collection for working with children: APN RSFSR, 1948.
Pisarev, A. Classical dance school 1976; Publishing house: L.: Art
Purtova T.V., Belikova A.N., Kvetnaya O.V. № Teach children to dance: a tutorial. Moscow: Vlados. 2003.
Smirnov, I.V. The Art of the Ballet Master 1986; Publishing house: M.: Enlightenment
Choreographic pedagogy: textbook. St. Petersburg: SPbGUP, 2006.
Aesthetic education by means of choreographic art / Ed. E.V. Konorova. M.: APN RSFSR, 1953.
Internet resources :
- http://www.
